Annuity (Present Value)

An annuity is a stream of equal withdrawals at regular intervals typically annually or semi-annually.
This is an example of a Geometric Sequence where all the numbers are multipled by a fixed amount (or common ratio).

Present Value of an Ordinary Annuity

An ordinary annuity is an annuity in which the first payment occurs at the end of the first period.
The following formula can be used to calculate the present value of an ordinary annuity.

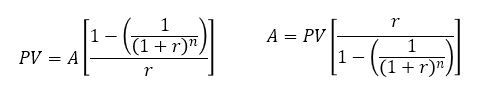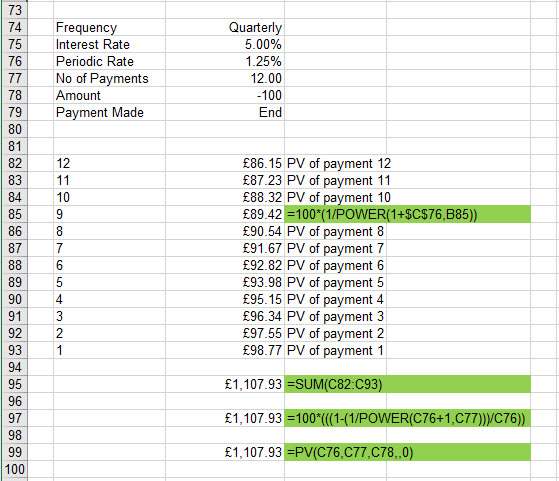
Example 1

What is the present value of an annuity that will pay £100 every 4 months for 3 years in an account that pays 5% interest every year compounded quarterly.

Example 2

Lisa would like to buy a flat and has taken out a 30-year mortgage for £300,000 at 3.2% interest, compounded monthly.
What will her monthly repayment be.

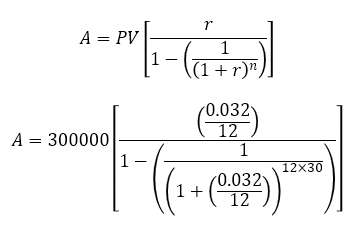Proof - Geometric Series

We know the formula for a single present value.

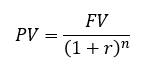Therefore the present value of an annuity would be the sum of all the individual present values.
This sum can be represented as a Geometric Series
To avoid any confusion with the letter 'r' the 'r' in the geometric series formulas has been changed to 'q'

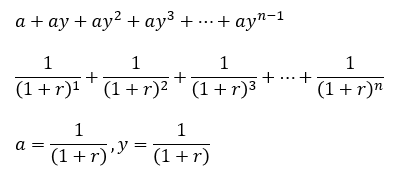We know the sum of the geometric series so we can make the necessary substitution.

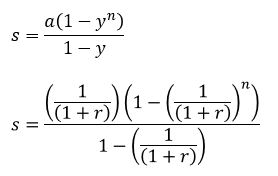Find a common denominator for the fraction underneath

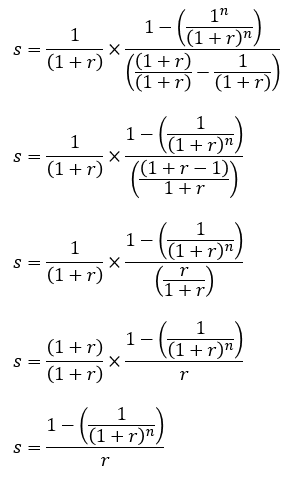Proof - Derived from FV

An alternative way of arriving at this equation is to substitute the value of FV in the equation.