Answers - Time Value of Money

Time Value of Money - Q1

If you have a semi-annual interest rate of 4.75% what is this annualised ?
This is equivalent to a nominal interest rate of (4.75 *2) compounded semi-annually.

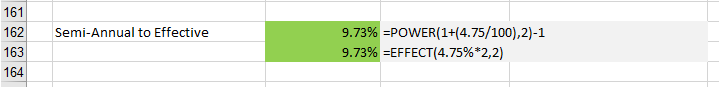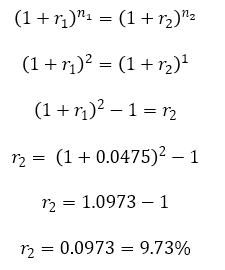Time Value of Money - Q2

Given an effective interest rate of 9.3%
What is the equivalent nominal rate compounded daily ?
N = (1 + 0.093)^(1/365) -1) * 365
N = 8.894%

Time Value of Money - Q3

An annual nominal interest rate of 10% compounded monthly actually means (10/12)% interest compounded every month.
If you borrow £100 for one year with an annual nominal rate of 10% compounded monthly, what is the total amount I pay back?
The amount of interest after one month is £0.83 (100 * (10/12)%)
The amount of interest after two months is £0.84025 ((100 + 0.83) * (10/12)%)
The amount of interest after three months is £0.84725 ((100.83 + 0.84025) * (10/12)%)
The amount of interest after four months is £0.85431 ((101.67025 + 0.84725) * (10/12)%)
The amount of interest after five months is £0.86143 ((102.5175 + 0.85431) * (10/12)%)
The amount of interest after six months is £0.86861 ((103.37181 + 0.86143) * (10/12)%)
The amount of interest after seven months is £0.87585 ((104.23324 + 0.86861) * (10/12)%)
The amount of interest after eight months is £0.88314 ((105.10185 + 0.87585) * (10/12)%)
The amount of interest after nine months is £0.89051 ((105.97770 + 0.88314) * (10/12)%)
The amount of interest after ten months is £0.89793 ((106.86085 + 0.89051) * (10/12)%)
The amount of interest after eleven months is £0.90541 ((107.75136 + 0.89793) * (10/12)%)
The amount of interest after twelve months is £0.91266 ((108.64929 + 0.90541) * (10/12)%)
The total amount after 1 year will be £110.46735
(100.00 + 0.83 + 0.84025 + 0.84725 + 0.85431 + 0.86143 + 0.86861 + 0.87585 + 0.88314 + 0.89051 + 0.89793 + 0.90541 + 0.91266)

Time Value of Money - Q4

Lets compare the following two interest rates
5 month (153 day) interest rate is 10.2% (this is a nominal interest rate compounded every 153 days)
91 day interest rate is 8.6% (this is a nominal interest rate compounded every 91 days)
The easiest way to compare them is to convert them both to effective interest rates (compounded annually)

E1 = (1 + ( 0.102 / (365/153) ) ^ (365/153) - 1
E1 = 10.5%

E2 = (1 + ( 0.086 / (365/91) ) ^ (365/91) - 1