Answers - Future Value

Future Value - Q1 - Simple Interest

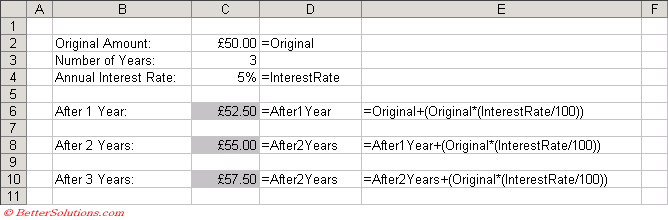
If £50 is invested for 3 years at an annual simple interest rate of 5% what is the total amount after 3 years.
Calculate the amount of interest every year and then add them to the present value

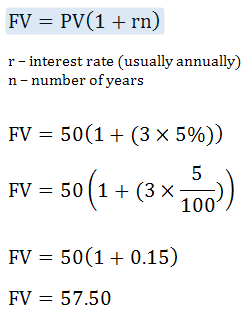Future Value - Q2 - Compounded Interest

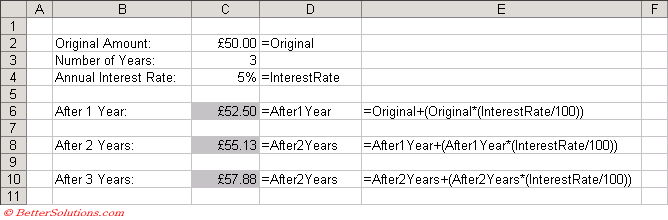
If £50 is invested for 3 years at an annual compounded interest rate of 5% what is the total amount after 3 years.
The total amount after 3 years will be £57.88.

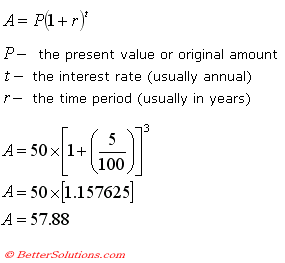Future Value - Q3 - Compounded Interest

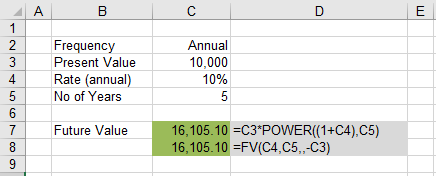
David invests £10,000 at an annual interest rate of 10% for 5 years.
What is the Future Value of this investment?
David would have £16,105.10

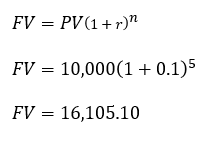Future Value - Q4

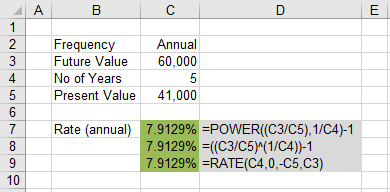
What annual interest rate would you need if you wanted to receive £60,000 after 5 years starting with an initial investment of £41,000?
The annual interest rate you need is 7.91%.

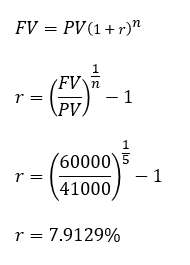Future Value - Q5 - Discount Factor

What is the 92 day discount factor if the interest rate for the period is 7.8%?
DF = 1 / ( 1 + ( r * ( d / 365) ) = 0.9807
Calculate the present value of $100 in 92 days time?
PV = 100 * 0.9807 = 98.07

Future Value - Q6

How much is £10,000 worth today if you received it in 4 years and the interest rate was 3.5%.
The discounted value of £10,000 is £9,662 over 4 years at an interest rate of 3.5%

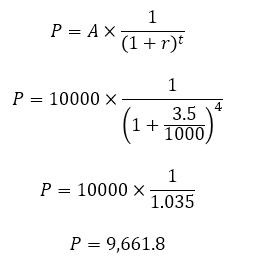Future Value - Q7

You have been offered one of the following two options, which one would you choose if interest rates are 4.2%
a) £5,000 in 3 years time
b) £6,000 in 5 years time

One way you can compare these two amounts is to discount the amount back to todays value.

You can use the NPV function to answer this question.
=NPV(4.2%,0,0,5000) = 4419.44
=NPV(4.2%,0,0,0,0,6000) = 4884.42

Future Value - Q8

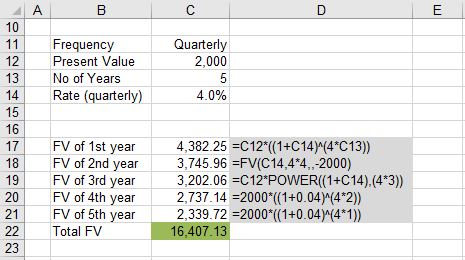
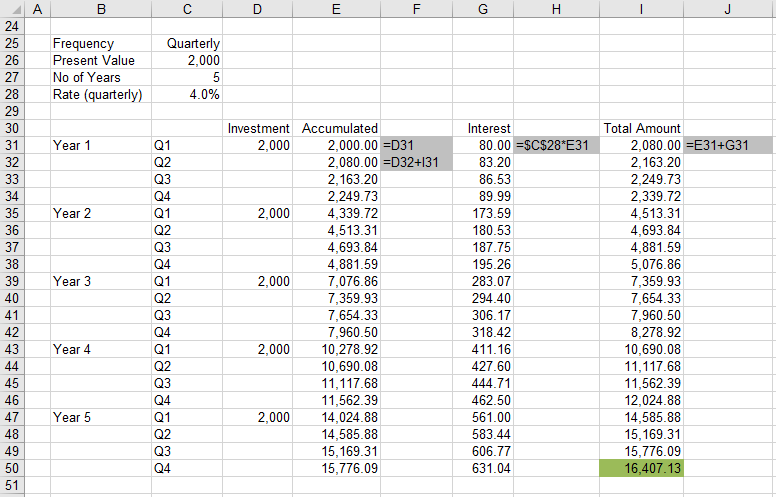
Michael invests £2,000 at a quarterly interest rate of 4% for 5 years.
Michael also adds an addition £2,000 at the end of each year.
What is the Future Value of this investment?
Michael would have £16,407.13

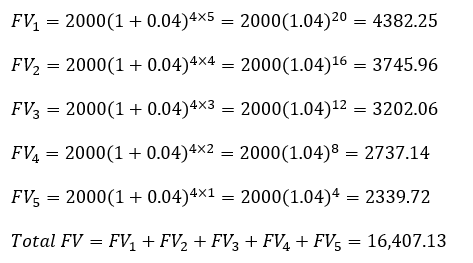Future Value - Q9

What is the Future Value of your investment if you deposit £1000 today plus £100 every month for the next 5 years with an interest rate of 3%.
FV = 1000*((1 + 0.03)^5)
FV = 1159.274

FV = 100*(((1+(0.03/12))^(12*5))-1)/(0.03/12)
FV = 6464.671